SUBHEAD: Revisiting a 1974 architectural theses project that laid out three Tiny Homes in a 8' wide 4D cube.
By Juan Wilson on 11 December 2019 for Island Breath -
(https://islandbreath.blogspot.com/2019/12/a-crooked-house.html)

Image above: Plan view of 8'x8' of Tiny Home and 8'x8' Tiny Yard. Home features single bed, kitchen counter with sink, work desk, shelving, cabinets, composting toilet with pass-thru panels connecting to other two homes. Click to enlarge.
Note: This is the initial posting of this work on our blog site and it will likely be upgraded and re-edited before it is finalized.
In the 1950's, when I was a teenager, I read short science-fiction story titled 'And He Built a Crooked House'. It is a science fiction short story by American writer Robert A. Heinlein, first published in Astounding Science Fiction in February 1941. It was reprinted in the anthology Fantasia Mathematica in 1958 and in the Heinlein collection The Unpleasant Profession of Jonathan Hoag in 1959.
The story is about a mathematically inclined architect named Quintus Teal who has what he thinks is a brilliant idea to save on real estate costs by building a house shaped like the unfolded net of a tesseract. The title is paraphrased from the nursery rhyme "There Was a Crooked Man". See (https://en.wikipedia.org/wiki/%22%E2%80%94And_He_Built_a_Crooked_House%E2%80%94%22).
The form of this unfolded 4D cube (or tesseract) took was a four story house of stacked cubes with four additional cubes wrapped around an upper floor.
At the time I had some interest in architecture and was fascinated by Heinlein's story. More than 20 year, while studying architecture at the Cooper Union in New York City I became interested in the representation of geometric objects through multidimensional space.
In 1973-74, as part of my Fifth Year Theses Project I investigated representing various 4D object concepts like "cube", "tube", "cup", "bottle" in drawing and model formats. One of my studies was a reevaluation of Heinlein's Crooked House architecture.
I decided to design a 4D regular cube that would be 8feet x 8feet x 8feet x 8feet. This hypercube would have there for have 8 8ft x 8ft x 8ft 3D volumes attached to one another as the "faces" of the 4D cube.
The eight 3D cube spaces include:
- One 8' cube of Earth with a small hyper-dense central rock cor providing One G of gravity to adjacent spaces. The earth cube is living soil providing needed organic materials and minerals to support plants.
- Three 8' cube Yards with grass and small tree rooted in the Earth cube. There is also a Welcome mat. The volume of the cube filled with breathable air.
- Three 8' cube Tiny Homes with a door (each facing a Welcome mat in one of the yards). Units also feature a wall with a window (facing an adjacent yard) a ceiling with skylight with access to the roof. There are two adjacent walls in each unit that abut the other two units.These otherwise blank walls each have a 16"x16" pass-through door that allow exchanging items between the other Tiny Home units without going outside. One pass-through door is red and the other green.
- One 8' cube of Sky A space that is above the roofs of the three living units and above the yards. At the center of the sky cube is a small sun-like sphere that provide light and energy for units and yards. At the center of the sphere is a small black hole 16" in diameter that is the only way in and out of this small universe.

Image above: Isometric views of 8'x8' elements, or parts, of 4D Tiny Home project include Home, Yard, Overhead Sky and Underfoot Earth.
The three Yard and Home cubes are arranged so that the six faces of the 8' Earth Cube supports the bottom of three Yards and three Tiny Homes.
It should be noted that the eight face cube volumes of a 4D hypercube are merely faces... much like the six faces of a empty cardboard box. It is usually what's inside the 3D space of the cardboard box that is the real prize.
Similarly the eight cube faces are merely the surface of the 4D cube. What is inside the 4D cube that is the real content... In this case maybe something to keep the Earth Cube fertile and the Yard Cubes breathable and moist and the Sky cube protective and a source of energy.
When the eight volumes of the tesseract are folded into the four dimensional hypercube the three Tiny Homes are abutted to one another and the three yards are contiguous.
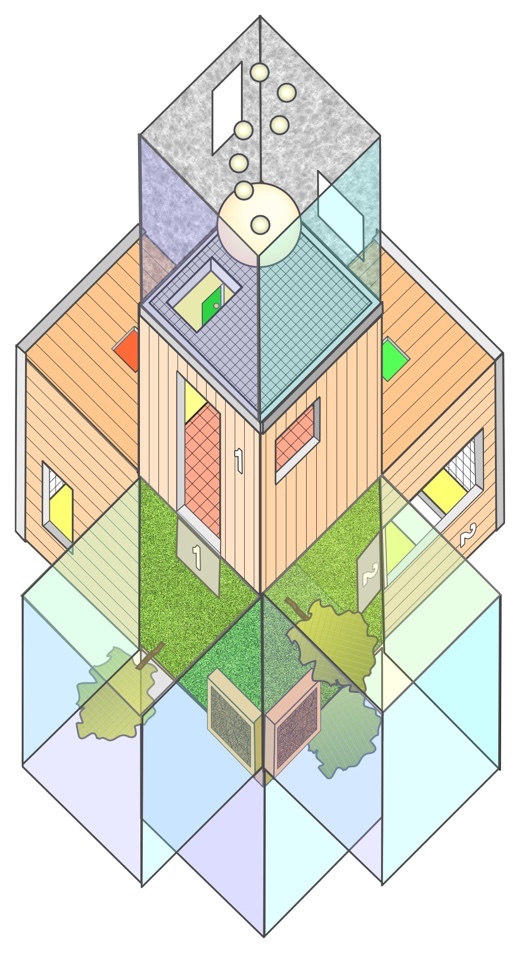
Image above: Isometric views of eight 8'x8'x8' elements of 4 dimensional Tiny Home project arranged into tesseract (4D unfolded cube analogous to a unfolded cardboard box.
The three Yard and Home cubes are arranged so that the six faces of the 8' Earth Cube supports the bottom of three Yards and three Tiny Homes.
It should be noted that the eight face cube volumes of a 4D hypercube are merely faces... much like the six faces of a empty cardboard box. It is usually what's inside the 3D space of the cardboard box that is the real prize.
Similarly the eight cube faces are merely the surface of the 4D cube. What is inside the 4D cube that is the real content... In this case maybe something to keep the Earth Cube fertile and the Yard Cubes breathable and moist and the Sky cube protective and a source of energy.

Image above: Two views, each showing four "sides" of the Hypecrcube's eight volumes when they are folded into the fourth dimension.
When the eight volumes of the tesseract are folded into the four dimensional hypercube the three Tiny Homes are abutted to one another and the three yards are contiguous. The two views above are analogous to looking at the opposite sides of a 3D dice, where you can only see the number of spots on 3 of the 6 sides of the dice. In the case of a 3D dice there are 8 unique views of 3 sides of the dice. In the case of a 4D cube there are 16 views of any four corner adjacent of the 8 volumes.
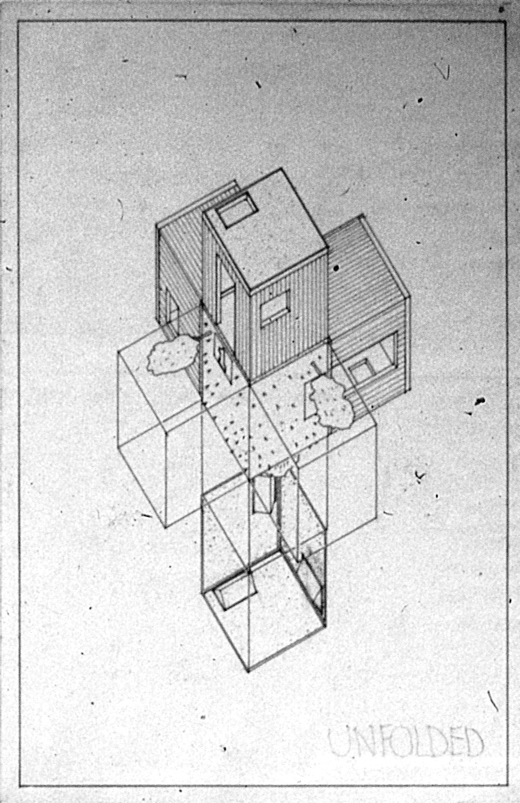
Image above: Pencil drawing from 5th year architectural thesis year at the Cooper Union by Juan Wilson in 1974. Isometric view of unfolded 4D Hypercube showing four of its eight volumes.

Image above: Pencil drawing from 5th year architectural thesis year at the Cooper Union by Juan Wilson in 1974. Isometric view from one side of the Hypercube showing four of its eight volumes.

Image above: Salvador Dali's 4D crucifixion titled "Corpus Hypercubus" from 1954. In his 1951 essay "Mystical Manifesto",Dali introduced an art theory he called "nuclear mysticism" that combined his interests in Catholicism, mathematics, science, and Catalan culture in an effort to reestablish classical values and techniques, which he extensively utilized in Corpus Hypercubus. From (https://en.wikipedia.org/wiki/Crucifixion_(Corpus_Hypercubus)).
By Juan Wilson on 11 December 2019 for Island Breath -
(https://islandbreath.blogspot.com/2019/12/a-crooked-house.html)

Image above: Plan view of 8'x8' of Tiny Home and 8'x8' Tiny Yard. Home features single bed, kitchen counter with sink, work desk, shelving, cabinets, composting toilet with pass-thru panels connecting to other two homes. Click to enlarge.
Note: This is the initial posting of this work on our blog site and it will likely be upgraded and re-edited before it is finalized.
In the 1950's, when I was a teenager, I read short science-fiction story titled 'And He Built a Crooked House'. It is a science fiction short story by American writer Robert A. Heinlein, first published in Astounding Science Fiction in February 1941. It was reprinted in the anthology Fantasia Mathematica in 1958 and in the Heinlein collection The Unpleasant Profession of Jonathan Hoag in 1959.
The story is about a mathematically inclined architect named Quintus Teal who has what he thinks is a brilliant idea to save on real estate costs by building a house shaped like the unfolded net of a tesseract. The title is paraphrased from the nursery rhyme "There Was a Crooked Man". See (https://en.wikipedia.org/wiki/%22%E2%80%94And_He_Built_a_Crooked_House%E2%80%94%22).
The form of this unfolded 4D cube (or tesseract) took was a four story house of stacked cubes with four additional cubes wrapped around an upper floor.
At the time I had some interest in architecture and was fascinated by Heinlein's story. More than 20 year, while studying architecture at the Cooper Union in New York City I became interested in the representation of geometric objects through multidimensional space.
In 1973-74, as part of my Fifth Year Theses Project I investigated representing various 4D object concepts like "cube", "tube", "cup", "bottle" in drawing and model formats. One of my studies was a reevaluation of Heinlein's Crooked House architecture.
I decided to design a 4D regular cube that would be 8feet x 8feet x 8feet x 8feet. This hypercube would have there for have 8 8ft x 8ft x 8ft 3D volumes attached to one another as the "faces" of the 4D cube.
The eight 3D cube spaces include:
- One 8' cube of Earth with a small hyper-dense central rock cor providing One G of gravity to adjacent spaces. The earth cube is living soil providing needed organic materials and minerals to support plants.
- Three 8' cube Yards with grass and small tree rooted in the Earth cube. There is also a Welcome mat. The volume of the cube filled with breathable air.
- Three 8' cube Tiny Homes with a door (each facing a Welcome mat in one of the yards). Units also feature a wall with a window (facing an adjacent yard) a ceiling with skylight with access to the roof. There are two adjacent walls in each unit that abut the other two units.These otherwise blank walls each have a 16"x16" pass-through door that allow exchanging items between the other Tiny Home units without going outside. One pass-through door is red and the other green.
- One 8' cube of Sky A space that is above the roofs of the three living units and above the yards. At the center of the sky cube is a small sun-like sphere that provide light and energy for units and yards. At the center of the sphere is a small black hole 16" in diameter that is the only way in and out of this small universe.

Image above: Isometric views of 8'x8' elements, or parts, of 4D Tiny Home project include Home, Yard, Overhead Sky and Underfoot Earth.
The three Yard and Home cubes are arranged so that the six faces of the 8' Earth Cube supports the bottom of three Yards and three Tiny Homes.
It should be noted that the eight face cube volumes of a 4D hypercube are merely faces... much like the six faces of a empty cardboard box. It is usually what's inside the 3D space of the cardboard box that is the real prize.
Similarly the eight cube faces are merely the surface of the 4D cube. What is inside the 4D cube that is the real content... In this case maybe something to keep the Earth Cube fertile and the Yard Cubes breathable and moist and the Sky cube protective and a source of energy.
When the eight volumes of the tesseract are folded into the four dimensional hypercube the three Tiny Homes are abutted to one another and the three yards are contiguous.

Image above: Isometric views of eight 8'x8'x8' elements of 4 dimensional Tiny Home project arranged into tesseract (4D unfolded cube analogous to a unfolded cardboard box.
The three Yard and Home cubes are arranged so that the six faces of the 8' Earth Cube supports the bottom of three Yards and three Tiny Homes.
It should be noted that the eight face cube volumes of a 4D hypercube are merely faces... much like the six faces of a empty cardboard box. It is usually what's inside the 3D space of the cardboard box that is the real prize.
Similarly the eight cube faces are merely the surface of the 4D cube. What is inside the 4D cube that is the real content... In this case maybe something to keep the Earth Cube fertile and the Yard Cubes breathable and moist and the Sky cube protective and a source of energy.

Image above: Two views, each showing four "sides" of the Hypecrcube's eight volumes when they are folded into the fourth dimension.
When the eight volumes of the tesseract are folded into the four dimensional hypercube the three Tiny Homes are abutted to one another and the three yards are contiguous. The two views above are analogous to looking at the opposite sides of a 3D dice, where you can only see the number of spots on 3 of the 6 sides of the dice. In the case of a 3D dice there are 8 unique views of 3 sides of the dice. In the case of a 4D cube there are 16 views of any four corner adjacent of the 8 volumes.

Image above: Pencil drawing from 5th year architectural thesis year at the Cooper Union by Juan Wilson in 1974. Isometric view of unfolded 4D Hypercube showing four of its eight volumes.

Image above: Pencil drawing from 5th year architectural thesis year at the Cooper Union by Juan Wilson in 1974. Isometric view from one side of the Hypercube showing four of its eight volumes.

Image above: Salvador Dali's 4D crucifixion titled "Corpus Hypercubus" from 1954. In his 1951 essay "Mystical Manifesto",Dali introduced an art theory he called "nuclear mysticism" that combined his interests in Catholicism, mathematics, science, and Catalan culture in an effort to reestablish classical values and techniques, which he extensively utilized in Corpus Hypercubus. From (https://en.wikipedia.org/wiki/Crucifixion_(Corpus_Hypercubus)).
Video above: Animations of rotating four dimensional cubes, tetrahedrons and Spheres by Eugene Khutoryansky. From (https://www.youtube.com/watch?v=eyuRLmCphHc).
.
No comments :
Post a Comment